Introduction
In the world of mathematics, quadratic equations 4x ^ 2 – 5x – 12 = 0 hold a special place. They’re like the puzzle pieces of algebra, offering insights into patterns and relationships that govern the physical world around us.
Today, we’ll dive into one particular quadratic equation: 4x^2 – 5x – 12 = 0. But don’t let the numbers intimidate you; we’ll unravel this equation together, step by step.
Quadratic equations often pop up in various fields, from physics to finance, and understanding how to solve them can unlock a host of problem-solving skills.
So, let’s embark on this journey of discovery as we demystify the process of solving quadratic equations, starting with this intriguing example. Ready? Let’s delve in!
What Is 4x ^ 2 – 5x – 12 = 0?
Our quadratic equation, 4x^2 – 5x – 12 = 0, is a mathematical expression that represents a specific relationship between a variable, in this case, ‘x’, and its square. Let’s break it down:

- 4x^2: This term signifies four times the square of the variable ‘x’. It’s the leading term of our quadratic equation.
- -5x: Here, ‘-5x’ represents five times the variable ‘x’, but with a negative sign attached. This term is the linear term of our equation.
- -12: The constant ‘-12’ is the last term in our equation. It’s a standalone number without any variable attached to it.
You May Also Like It
Trendzguruji.me – Computer, Cyber, Awareness, SEO, Health & Beauty Guide
TrendzGuruji.me Cyber Insights Hub
“What’s Up with Emerald Gems? Let’s Talk”
Why is Understanding Quadratic Equations Important?
Quadratic equations play a crucial role in mathematics and have wide-ranging applications in various fields. Here’s why understanding them is essential:
- Foundation of Algebra: Quadratic equations serve as a fundamental building block in algebraic problem-solving. Mastering quadratic equations enhances overall algebraic proficiency, paving the way for tackling more complex mathematical concepts.
- Real-World Applications: These equations model numerous real-world phenomena, such as the trajectory of a projectile, the shape of a parabolic mirror, or the behavior of springs and pendulums. Understanding quadratic equations enables us to analyze and predict these phenomena accurately.
- Problem-Solving Skills: Solving quadratic equations requires critical thinking and problem-solving skills. By learning different methods to solve them, such as factoring, completing the square, and using the quadratic formula, individuals develop analytical skills applicable beyond mathematics.
- STEM Fields: Quadratic equations are prevalent in science, technology, engineering, and mathematics (STEM) disciplines. Proficiency in solving them is essential for success in fields like physics, engineering, economics, and computer science.
- Optimization and Modeling: Many optimization problems in economics, engineering, and management involve quadratic equations. Understanding how to formulate and solve these equations is crucial for optimizing processes and making informed decisions.
- Historical Significance: Quadratic equations have a rich historical background, dating back to ancient civilizations like Babylon and Greece. Studying quadratic equations allows us to appreciate the contributions of mathematicians throughout history and understand the evolution of mathematical thought.
Step-by-Step Guide to Solving 4x^2 – 5x – 12 = 0

Step 1: Understand the Quadratic Equation
- First, familiarize yourself with the quadratic equation 4x^2 – 5x – 12 = 0. Identify the coefficients: ‘a’ is 4, ‘b’ is -5, and ‘c’ is -12. Knowing these values will guide you through the solution process.
Step 2: Calculate the Discriminant
- The discriminant, denoted by Δ (Delta), is a crucial determinant of the nature of the solutions. Calculate it using the formula Δ = b^2 – 4ac. For our equation, Δ = (-5)^2 – 4(4)(-12).
Step 3: Determine the Nature of the Solutions
Based on the value of the discriminant:
- If Δ > 0, there are two distinct real solutions.
- If Δ = 0, there is one real solution (the roots are repeated).
- If Δ < 0, there are two complex solutions (conjugate pairs).
Step 4: Substitute Values and Solve
- Plug in the values of ‘a’, ‘b’, and ‘c’ from your equation into the quadratic formula. Calculate the solutions for ‘x’ using both the plus and minus signs.
Step 5: Simplify
- Perform the necessary arithmetic operations to simplify the expressions under the square root and within the fraction. Ensure your solutions are in simplest form.
Advantages:
- Versatility: Quadratic equations are versatile mathematical tools applicable in various fields such as physics, engineering, economics, and computer science. They provide a framework for modeling and analyzing real-world phenomena.
- Predictive Power: By solving quadratic equations, we can make predictions and projections about the behavior of systems and processes. This predictive power is invaluable in decision-making and optimization tasks.
- Problem-Solving Skills: Working with quadratic equations enhances problem-solving skills, fostering logical thinking and analytical reasoning. The process of solving quadratic equations develops critical mathematical skills applicable in many areas of life.
- Historical Significance: Quadratic equations have a rich historical background, dating back to ancient civilizations. Studying quadratic equations allows us to appreciate the contributions of mathematicians throughout history and understand the evolution of mathematical thought.
Disadvantages:
- Complexity: Quadratic equations can become complex, especially when dealing with equations that don’t factor easily or have irrational solutions. This complexity can pose challenges for students and require advanced problem-solving techniques.
- Multiple Solutions: Quadratic equations can have multiple solutions, including real, imaginary, or repeated roots. Interpreting and analyzing these solutions accurately may require additional effort and mathematical understanding.
- Limited Applicability: While quadratic equations are powerful tools, they may not always accurately model certain real-world phenomena. In some cases, more sophisticated mathematical models or techniques may be required for precise predictions and analysis.
- Dependency on Initial Conditions: The solutions to quadratic equations can be sensitive to changes in the coefficients or initial conditions of the equation. Small variations in these parameters can lead to significantly different outcomes, requiring careful consideration in practical applications.
What is a quadratic equation?
A quadratic equation is a mathematical expression involving a variable raised to the power of two (usually written as x^2) and other terms with lower powers of the variable. It’s like a special puzzle in math!
How do I recognize a quadratic equation?
Look for the x^2 term! If you see something like 2x^2 – 3x + 1 = 0, that’s a quadratic equation. It’s all about that x^2 term.
Why are quadratic equations important?
Quadratic equations help us understand lots of real-life stuff, like how things move through the air or how prices change. They’re like magic math tools for predicting and understanding the world around us!
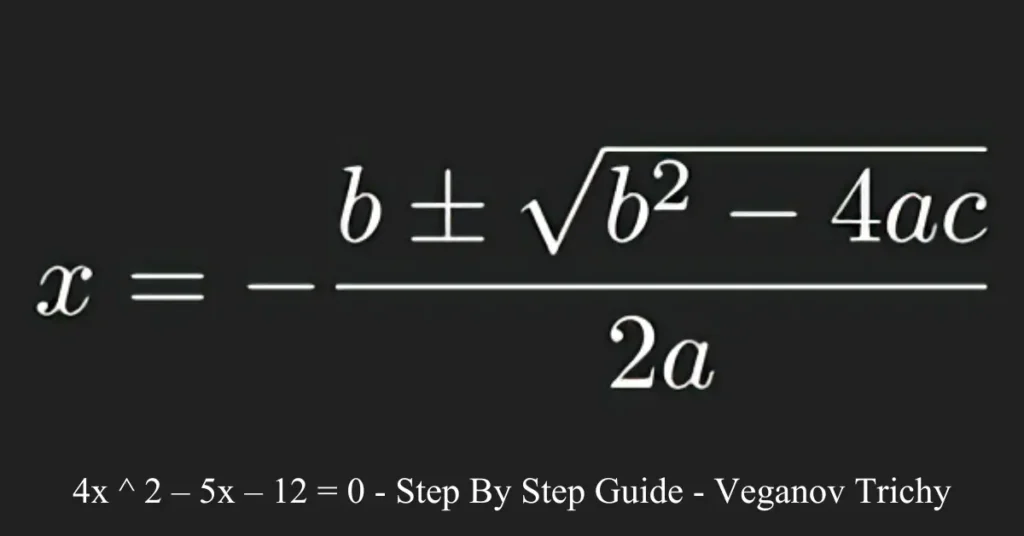
How do I solve a quadratic equation?
There are a few ways, but one common method is using the quadratic formula: x = (-b ± √(b^2 – 4ac)) / (2a). Just plug in the numbers from your equation and follow the steps!
What if my quadratic equation doesn’t factor nicely?
No worries! You can still use the quadratic formula mentioned earlier. It’s like a special formula that always works, no matter how tricky the equation.
Conclusion
Quadratic equations are like powerful tools in the world of math. They help us solve puzzles, predict outcomes, and understand how things work in real life.
While they might seem tricky at first, with practice and patience, anyone can learn to tackle them. So keep exploring, keep learning, and remember, math is all about having fun and discovering new things!
Bonus Point
- Graphical Representation: Quadratic equations can be graphed as parabolas, which have fascinating properties like symmetry and vertex points. Exploring these graphs visually can deepen your understanding of quadratic equations.
- Applications in Computer Graphics: Quadratic equations play a significant role in computer graphics, helping create realistic images in video games, animations, and simulations. Understanding quadratics can open doors to careers in digital media and entertainment.
- Connection to Other Math Concepts: Quadratic equations are closely related to other mathematical concepts, such as functions, polynomials, and complex numbers. Mastering quadratics can improve your understanding of these interconnected topics.
- Historical Significance: Quadratic equations have a rich history, with roots tracing back to ancient civilizations like Babylon and Greece. Exploring the historical development of quadratics can provide insight into the evolution of mathematical thought.
- Extensions and Generalizations: Beyond the standard quadratic equation, there are many extensions and generalizations, such as quadratic inequalities, systems of quadratic equations, and higher-degree polynomials. Delving into these topics can broaden your mathematical horizons.
- Problem-Solving Challenges: Quadratic equations often appear in math competitions and problem-solving challenges. Sharpening your skills in solving quadratic equations can prepare you for tackling more complex mathematical problems.
- Real-World Projects: Engage in hands-on projects that involve quadratic equations, such as designing a roller coaster, analyzing the motion of projectiles, or optimizing business processes. Applying quadratic equations to real-world scenarios enhances your practical problem-solving abilities.
- Exploration with Technology: Use graphing calculators or mathematical software to explore quadratic equations dynamically. Experiment with different coefficients and see how they affect the shape and behavior of the quadratic graph.
- Collaboration and Discussion: Join math clubs, forums, or study groups to collaborate with others on quadratic equation problems. Sharing ideas and strategies can deepen your understanding and foster a sense of community in your mathematical journey.
- Fun Facts: Did you know that the ancient Greeks used geometric methods to solve quadratic equations? Or that the word “quadratic” comes from the Latin word “quadratus,” meaning “square”? Exploring fun facts about quadratics can make learning about them even more enjoyable!
You May Also Like It
Instagram Par Follower Kaise Badhaye With Can Follow Website – Veganov Trichy
1000+ Free Instagram Story Views In 2024 – Veganov Trichy
How To Increase Followers On Instagram With TakipciGir New Update 2024